Everything You Always Wanted to Know About Alpha Shapes But Were Afraid to Ask

What are alpha shapes?

The concept of alpha shapes is an approach to formalize the intuitive notion of "shape" for spatial point sets. The Alpha shape is a concrete geometric concept which is mathematically well defined: it is a generalization of the convex hull and a subgraph of the Delaunay triangulation. Given a finite point set, a family of shapes can be derived from the Delaunay triangulation of the point set; a real parameter, "alpha," controls the desired level of detail. The set of all real alpha values leads to a whole family of shapes capturing the intuitive notion of "crude" versus "fine" shapes of a point set.

Here is an example for different values of alpha. Notice the second picture which displays the boundary of the point set's convex hull.

Formal definition

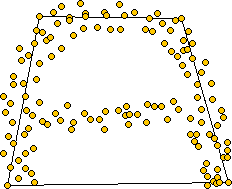
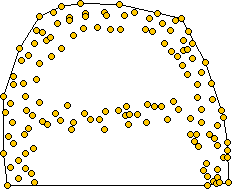
First, let us define a generalized disk of radius 1/alpha to be the following:

• If alpha > 0, it is an ordinary closed disk of radius 1/alpha
• If alpha = 0, it is a halfplane
• If alpha < 0, it is the complement of a closed disk of radius -1/alpha

Then, given a set of points and a specific value for alpha, we construct the alpha shape graph in the following way:

1. For each point Pi in our point set, we create a vertex Vi.
2. We create an edge between two vertices Vi and Vj whenever there exists a generalized disk of radius 1/alpha containing the entire point set and which has the property that Pi and Pj lie on its boundary.

Applet Instructions

• Insert & drag points using the left mouse button
• Delete a point using the right mouse button
• Try different values for alpha by gliding the bottom cursor
• The top-left corner displays the generalized disk which corresponds to the current value for alpha
• Turn on or off the various checkboxes in order to display other informations
• Try out the demo!! (you may have to wait a few seconds before it starts due to file downloading).

Comments & Suggestions

• If you have any comment concerning this applet, or if you are interested in having a copy of the undocumented & comment-free source code, just send email to banzai.

Other Alpha Shapes Links

• NCSA's Alpha shapes software
• Ken Clarkson's code for computing alpha shapes
• References on alpha shapes

Back to my home page